Hooke's Law

Hooke’s Law – Computer Applications Assignment 1

Hooke’s Law is the law of elasticity discovered by Robert Hooke in 1660, which states that, for relatively small deformations of an object, the displacement or size of the deformation is directly proportional to the deforming force or load. ⁽¹⁾

Deformation of a solid is caused by a force that can either be compressive or tensile when applied in one direction. Compressive forces try to compress and object while tensile ones try to tear it apart. We can study these effects by looking at what happens when you compress or extend a spring. ⁽²⁾

The equation which relates deformation to load is written as;

F=-kx (Equation 1⁽³⁾)

In equation 1, F is equal to the force applied; k is the spring constant of the material and x is the amount of displacement observed.

When plotting a graph of load against displacement, a linear relationship is observed. 

(Figure 1 ⁽²⁾)

However, Hooke’s Law is only obeyed until a certain point. If a great enough force is applied to the material, it will deviate from the linear relationship previously observed. The point where Hooke's law stops being obeyed is known as the elastic limit. When looking at the trend line on a graph, it will start to curve and this trend is shown best in figure 4. When this point is passed, the material will no longer return to it's original shape. Each material will deviate from this relationship differently and this is how materials are classified. ⁽²⁾

 

                     (Figure 2 ⁽²⁾)                                                           (Figure 3 ⁽²⁾)

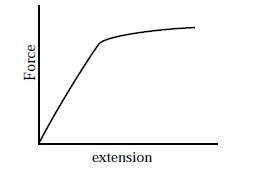
Figure 2 shows the relationship between force and extension for a strong, brittle material. There is very little extension for a large force, but the material suddenly breaks and fractures. It is defined as being brittle because the material fractures instead of bending. An example of a brittle material is glass. ⁽²⁾

Figure 3 shows the relationship between force and extension for a plastic material. The material undergoes a large deformation with only a little force applied. The linear relationship of Hooke’s Law only lasts for a short time. It is defined as being plastic because it is more likely to bend than to fracture. ⁽²⁾

(Figure 4 ⁽²⁾)

Figure 4 shows the relationship between force and extension for a ductile material. The material shows the behaviour of a plastic material, after Hooke’s Law has been exceeded, over a range of forces before fracturing.  Ductility is the ability of a material to be stretched into a new shape without breaking. An example of a ductile material is aluminium. ⁽²⁾

Most Hooke’s Law experiments are carried out by hanging weights onto a spring and recording how much they extend by. Here is a video demonstrating how to set up and conduct a Hooke's Law experiment.

Stretching a Spring⁽⁴⁾

Figure 5 shows how when the force acting on the spring doubles, the spring's extension also doubles.

(Figure 5 ⁽⁵⁾)

Here are a few videos which explain Hooke’s Law and what to expect from a force and displacement graph.

Kahn Academy - Intro to Springs and Hooke's Law⁽⁶⁾

High School Physics - Springs and Hooke's Law⁽⁷⁾

The following results were obtained from a Hooke’s Law experiment.

x	y1 (Material 1)	y2 (Material 2)	z (Material 3)
1	3	2.2583	2.375
2	4.5	4.3166	9.375
3	6	6.3749	28.375
4	7.5	8.4332	65.375
5	9	10.4915	126.375
6	10.5	12.5498	217.375
7	13	14.6081	344.375
8	14	16.6664	513.375
9	15	18.7247	730.375

(Table 1)

In table 1, x is the amount of force applied in Newtons, and the values shown in the material columns show the amount of deformation in the material in mm.  When plotting materials 1 and 2 on a graph, a trend becomes evident. 

(Figure 6)

Figure 6 shows, that both materials have a linear relationship with force and extension. This means that Hooke’s law is being obeyed throughout all points of the experiment. This also implies that, once the load is removed, the material will return to its original shape. Also, as the relationship is linear, they both follow equation 4 which is the equation of a straight line.

Y=mx+c (Equation 4)

Using equation 4, it is possible to work out the gradient of the slope and also the y intercept. In equation 4, the gradient of the slope is equal to m and the y intercept is equal to c. In this case, the gradient is a constant and is also equal to the spring constant of the material itself. Equation 2 shows the spring constant for material 1 as being 1.5583 Nm^-1. Equation 3 shows that the spring constant for material 2 as being 2.0583 Nm^-1.

The y intercept shows the force applied when displacement is zero. It is to be expected that the y intercept for Hooke’s law will be equal to zero. That is to say, that when there is zero force applied, zero deformation is observed. However, as can be seen from equations 2 and 3, there is a value of 0.2 and 1.375 respectively. These values obtained for the y intercept is due to choosing a line of best fit from the data collected.

It is also possible to use the graph in figure 6 to show the point where the force-extension relationships for both materials intersect each other. It is at this point where both materials will extend by the same amount when the same force is applied to them. According to the graph this occurs at roughly 2.3N. This can also be attained by using the laws of simultaneous equations to equations 2 and 3 in the following way.

First arrange the equations together as equation 2 is equal to equation 3 to get equation 5;

2.0583x + 0.2 = 1.5583x + 1.375 (Equation 5)

Next, make x the subject of equation 5 to get equation 6;

2.0583x – 1.5583x = 1.375 – 0.2 (Equation 6)

Next, do the subtraction on both sides of the equation to get equation 7;

0.5x = 1.175 (Equation 7)

Finally divide both sides of equation 7 by 0.5 to leave equation 8;

X = 2.35 N (Equation 8)

The value which appears on figure 6 is very close to the value obtained in equation 8. It is not identical due to the graph in figure 6 not being presented in enough detail to gain an exact reading of where the intersection takes place.  

Figure 7 shows the results for material 3 plotted on a force and extension graph. It shows that the values obtained do not show a linear quality. As force is applied, the amount of deformation is greater than to be expected of a linear relationship. From this we can determine that material 3 does not follow Hooke’s Law and can be categorised as a plastic material. This can be verified when comparing figure 6 to figures 1, 2 and 3.

(Figure 7)

References

1.       Unknown. (2012). Hooke's Law. Available: http://www.britannica.com/EBchecked/topic/271336/Hookes-law. Last accessed 12th Nov 2012.

2.       Unknown. (6th Oct 2009). What Is Hooke's Law?. Available: http://engineers4world.blogspot.co.uk/2009/11/hookes-law.html. Last accessed 12th Nov 2012.

3.       Unknown. (2012). Determine The Spring Constant. Available: http://www.4physics.com/phy_demo/HookesLaw/HookesLawLab.html. Last accessed 12th Nov 2012.

4.       QuantumBoffin. (7th Nov 2009). Stretching a Spring. Available: https://www.youtube.com/watch?v=OHyfoM2vIUs&feature=related. Last accessed 13th Nov 2012.

5.       Nave, R. (Unknown). Elasticity - Hooke's Law. Available: http://hyperphysics.phy-astr.gsu.edu/hbase/permot2.html. Last accessed 13th Nov 2012.

6.       Fullerton, D. (29th Nov 2011). Springs and Hookes Law. Available: http://www.youtube.com/watch?v=6MhaPzGxfV8&feature=related. Last accessed 13th Nov 2012.